applicationsite.blogg.se

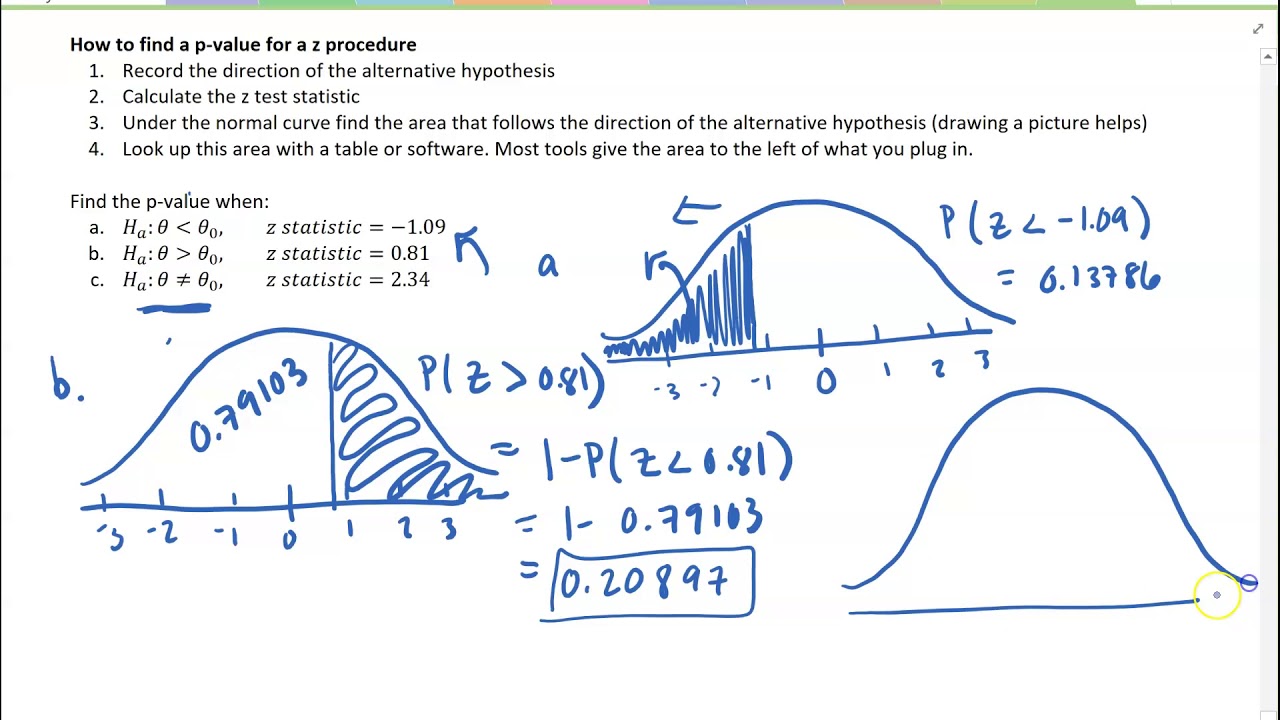
Z-test for sampling distribution is used to determine whether the sample mean is statistically different from the population mean. Recall that the sampling distribution is defined as the distribution of all the possible samples that could be drawn from a population. Note that the sampling distribution is used in the hypothesis testing technique known as Z-test. When considering the sampling distribution, Z-score or Z-statistics is defined as the number of standard deviations between the sample mean and the population mean (mean of the sampling distribution). Z-score or Z-statistics for Sampling distribution to perform Z-test In the same way, Z=-1 represents that the observation is 1 standard deviation away from mean in the negative direction. Note that Z = +1 represents that the observation is 1 standard deviation away from mean in the positive direction. The figure below represents different values of Z-scores. When the mean and standard deviation of a data set are known, it is easy to convert them into Z-score for that particular sample or population. The process of converting raw observations into Z-score is also called as standardization or normalization. Observation 45 is -1.5 standard deviation away from the mean 90. The observation X = 45 will have Z-scores as follows: Suppose, the mean of data points in a sample is 90 and the standard deviation is 30. Let’s take an example to understand z-score calculation better. Σ is the standard deviation of the observations in the sample X̄ is the mean of the observations in the sample Alternatively, when defined for population, Z-score can be used to measure the number of standard deviations by which the data points differ from the population mean. When considering a sample of data, Z-score is used to measure the number of standard deviations by which the data points in the sample differ from the mean. The hypothesis tests where Z-statistics get used is one-sample Z-test and two-samples Z-test.Īdditionally, z-score at different confidence intervals can be used to estimate the population mean based on a given sample or difference in the population means based on two different samples. Z-score for sampling distributions: In case of sampling distribution, the goal is to perform Z-test and find the value of Z-score or Z-statistics for hypothesis testing and rejecting the null hypothesis or otherwise.Z-score for sample data: In case of sample data, the objective is to find number of standard deviations an observation is away from the sample mean.The Z-score formula differs when considering the sample data, or, when considering sampling distributions with an end goal of whether finding the deviation from mean, or, performing hypothesis testing respectively. Z-score / Z-statistics Concepts & Formula

Z-score for Estimating Population Mean/Proportion.Z-score or Z-statistics for Sampling distribution to perform Z-test.Z-score / Z-statistics Concepts & Formula.